DOI: 10.24308/iass-2014-041

Technological Educational Institute of Epirus, Arta, Greece

papadaki@teiep.gr

Abstract

While drawing, children, as well as artists and scientists are energetically searching, choosing, simplifying, abstracting, analyzing, composing, completing, correcting, comparing, demarcating. All these processes form the way that the human brain elaborates cognitive material in every level. Children’s and adults’ drawings can be seen as critical tools and discovery processes, valuable perception aids, visual thinking mechanisms and routes to knowledge (Papadaki 2013). Both children and adults use the same resources (lines, shapes, orientation etc.) in order to realize meaning. These semiotic acts “should be seen as part of an intertextual chain… as stages in an ongoing dialogic process” (Van Leeuwen 1998:275). Artists, scientists and children alike construct knowledge as they deploy meaning across different modes and combine them in image ensembles (Kress 2003:141).

This paper provides the framework of a larger research which is still in development and tries to interpret children’s understanding of space by examining children’s drawings of spaces having in mind artistic and scientific spatial drawings. The research focuses on drawings made by children between 4 and 12 years old (kindergarten and elementary school).

1. Space in art drawings

Space is a major, as well as a complex concern in art and it has been so throughout the history of art. Some artists, such as Frank Stella, see the creation of space as theprincipal goal of art. From Renaissance art that tried to depict three-dimensional space using perspective to 19^th century art of Turner and the Impressionists and further on to 20^th century experiments of spatial and space-time representations much has changed. Art has never stopped investigating the concept of space. Abstract explorations of space are nowadays common. Space is implied through the examination of surface, texture and color. Conceptual ideas about space are implied through optical impressions.

2. Space in science drawings

a.  Mathematics, physics

Dictionaries define mathematical space as a set of elements or points satisfying specified geometric postulates or the infinite extension of the three-dimensional region in which all matter exists.

In scientific drawings a topological statement or drawing determines a spatial relationship, like something that is included in something else. Geometry is the study of spatial forms. It involves representation of shape, size, pattern, position and movement of objects in the three-dimensional world, or in the mind of the learner. It investigates three-dimensional objects and two- dimensional shapes as well as the concepts of position, location and movement.

b.  Architecture

A challenge in terms of representation, architecture relies on drawings. The form of the three-dimensional building appears as lines on the two-dimensional paper. Form encloses space and by doing so gives space its shape (Spankie 2009:12). For Adolf Loos, the creation of space should be driven primarily by the effect that the designer wishes to exert upon the spectator. Through drawing, the architect externalizes initial thoughts in sketch form, tests ideas, evaluates solutions and solves problems before they occur. He/she speculates on alternatives. The architect uses drawing as a critical tool (Haralambidou 2007:234) or a set of instructions. He/she accepts standard representational conventions, the language of drawing, in order to firstly observe and record and secondly as a way of "thinking aloud”. For architecture, drawing generates space.

3.  Children’s drawings of space

How space is perceived in the children minds is a much studied subject in developmental psychology. A child’s drawing of three-dimensional space on a two-dimensional piece of paper is a puzzling achievement. Drawing appears to be a useful tool in fostering mental model building with respect to the representation of spatial relations (Leopold & Leutner 2012:9). The process of drawing makes topological relations among components explicit and therefore helps the learner to identify relations among the components of a displayed object (Larkin and Simon 1987).

We live in a three-dimensional world and should, therefore, be able to understand and depict depth, continuity, surrounding, order, enclosure, scale. Drawing helps children “represent features and relations structurally analogous to those of the reference objects” (Leopold & Leutner 2012:2).

The drawings for this research are made by children aged 4-12. There are different phases in the development of sign processes in children, as the sign system evolves during the process of aging (Sebeok 1977). Based on Piaget’s theory on mental development in children, Krampen (1991) speaks about “developmental semiotics”. A child begins to produce meaning by indexical signs based on present objects (perception and perceptual activity). At this stage, children start drawing simple schemes—the lowest level of semiotic structure or mere foregrounding of a sign (Krampen 1991). As they grow older, they go on to producing meaning by symbols (imagination). They now draw more complex functional schemes—second level of semiotic structure or syntactic information (Krampen 1991). Finally, through high degree of abstraction, the child comes to produce meaning using signs. The third level of semiotics entails context. For Peirce (1965:143) the relevant phases are named iconic, indexical and symbolic, while Bruner (1966) talks about the enactive, the iconic and the symbolic phase. Luquet (1927) has proposed five stages in children’s development: scribbling, fortuitous realism, failed realism, intellectual realism and visual realism. Children’s drawings are considered a type of symbol appearing in the transition between symbolic play and imagination (Krampen 1991:22). Matthews (1984:1) suggests that “the child builds iconography on a substratum of symbolizations” at a very young age, before and during the “scribbling stage”.

Following the many attempts of a boy named Christos to draw a car from the age of three until the age of six, will show the three stages of drawing. The car is for Christos of outmost importance, because he travels very often and in addition, it connects his family (his father works in a different city than the one Christos lives in and the car either transfers him to his father or brings his father home).

At the age of three, Christos starts from the experiential perception regarding his relation with the car, but he also deals with it as a thing in as such, as no element of the external surroundings are visible in the picture (figure 1). He does not see it as an external schema — the internal is equally important. In this drawing, the car, despite the fact that is a very nice abstraction, has not taken the form of a symbol.

Figure 2, drawn at the age of 4, is a rational approach. It focuses on functional elements, like wheels, seat, steering wheel. The convertible schema helps him with his goal and the linear representation helps him grasp the meaning. The indication of the environment, a line, shows his try to perceive functionality.

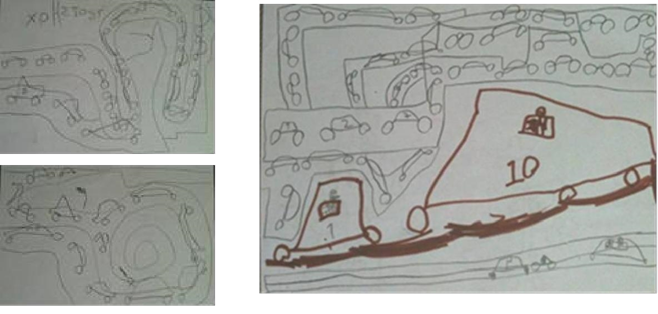Figure 4: different drawing instances of racing cars drawn by Christos at the age of 5+

Figure 4 shows different drawing instances of cars drawn by Christos at the age of 5,5. The numbers on the cars denote racing cars. The third drawing is the final drawing of a series of drawings concerned with car races. Christos considered it as the best and completed one, so he did not have to draw any other. When asked why this was the best one he answered that the biggest car in the drawing is the leader of the race (shaped almost as a triangle because it is the most dynamic and the faster one) and that Christos himself is standing near the finishing line and he is going to be the first who sees the winner. The biggest car is therefore the one that is closer to the spectator. In this drawing, Christos managed to give the illusion of space, using techniques such as placement on paper, size, color and detail. The closest car is colored with a darker, warm color, it is bigger than all the others and details such as the driver and the number on it are shown perfectly clear. The second car of the race still has these characteristics (except that it is smaller and less of a triangular shape), but all the others are not colored, small and the details are not visible. We, the spectators, cannot see them, as they are too far away. Christos has managed to show depth/space.

4.  The research

About 1000 children from 12 schools in Greece were asked to draw four images of spaces: their room, their house, a map and a maze. The research took into account two important issues:

-        the importance of the socio-cultural influences (see Alland 1983, Arnhein 1954, 1969, Golomb 1992, Goodnow 1977, Kellogg 1970) that shape children understanding and depiction of space, as well as their understanding of the value of drawing

-        the importance of the curriculum in the children familiarization of the various drawing techniques

The sample for the research was chosen randomly but includes children both from big cities and from small villages, both in mainland Greece and in islands, in order to examine children from various Greek socio-cultural settings, following both the standard school curriculum and the enlarged zone curriculum, which includes drawing, PC learning, theatrical education, foreign language and music.

The children that took part at this research were between the ages 4-12. The reasons for the selection of this wide age-group were many: the examination of the developmental direction (both physical and mental growth) in spatial presentation of children drawings and the acquisition of specific techniques of spatial representation depending on age, school curriculum and socio-cultural influences, the possibility of following universal laws of drawing space (learned from art and/or science) and leaving aside socio-cultural influences as children grow older.

For the purposes of this paper, as well as for the sake of economy, and as the research is still in development (in the process of gathering the children drawings from the island of Crete and mainland Epirus), I will focus on the presentation of three main issues that seem interesting about children’s use of the semiotic system of drawing in order to present space: a) organization/arrangement of objects on the paper provided, b) depth (as an implication of space) and c) socio-cultural differences between the children living in a big city and the children living in a village. I will use as the sample of this small study the 400 drawings I have gathered up to this point. These drawings were made by children living in northern Greece, in the city of Thessaloniki and a village nearby called Koufalia.

5.  The first findings

The notion of space implies a certain organization and arrangement of things. Agreeing with Golomb, there are two main compositional schemes in children drawing, which are noticeable in the sample gathered do far:

1.     Alignment of items in a grid like fashion along both horizontal and vertical axes and

2.     Centering strategies, which organize items around a pictorial center (Golomb 1992:166).

The youngest children of the sample drew with vivid colors and much enthusiasm the first two themes: their room and their house. There were many similarities in the drawings. The vast majority of the children (almost 80%) in the space provided for drawing their room drew a bed. The bed represented their room, as the main reason they stayed in their room was sleep. The bed was usually drawn as seen from a point above it (borrowing the Japanese bird-eye view technique). Young children use the biggest abstractions, the objects are thought of as being important in themselves, without any reference to their surroundings.

Figure 5a, b and c: Drawings of children aged 4-5 (elementary school). Theme: my room

Figure 6a, b and c: Drawings of children aged 4-5 (elementary school). Theme: my room

Most of the children placed the objects of their drawings in horizontal and vertical axes (defined by the space on the paper provided for their drawings) and only a smallminority organized the items around a pictorial center. There were no noticeable differences between the children living in the village and the children living in the big city, as far as the drawings of their rooms were concerned.

The case was similar with the drawings of the children’s houses. Almost all the children drew on a baseline (the one marking the bottom end of the provided space) the same square with the triangle on top which they have learned to decipher as the “home”. There were two interesting tendencies regarding the house drawings: a. many children drew their houses as transparent, using the X-ray technique, so that the spectator could peep into the house (figure 7c) and b. many children drew figures of their mother and father beside or in the house, trying to imply that their house is where their mother and father are (figure 7a and 7b). There is no sense of scale in the drawings: the human figures are almost the same size as the house. Some children living in Thessaloniki drew blocks of flats (figure 7d), while the children living in Koufalia drew only detached houses.

Figure 9a, b and c: Drawings of mazes by children aged 4-5

Figure 10a, b, c, d, e, f: Drawings by first grade pupils. Theme: my room

The drawings collected from the first grade are much different. The children drew their rooms following at a greater extent centering techniques (figures 10a, 10d and 10e). More furniture and personal items appear in the children’s rooms (figures 10b, 10c, 10d and 10e). As the first grade marks the first year that children have schoolhomework, the desk is the main furniture in the drawings of the children’s rooms.

Regarding the drawings of the children’s houses, distinguishing features of the houses are used in order to stress the uniqueness of each specific house (figures 11b and 11c). A furnished balcony and a dog nearby (figure 11c), a staircase in the house and a tractor outside (figure 11b) characterizes the place as unique, determines its identity. It is now recognizable by everyone as a specific child’s family home. The underlying of the uniqueness of a house seemed especially important for the children living in the village. When asked where they live, children living in the big cities answered with a street name and a number. Children living in villages, however, gave a descriptive answer: “at the stone house at the end of the street”, or “at the green house behind the white fence”.

The first attempts to show depth—using the technique of perspective—are visible (see figures 10c, 10f and 11a): children draw the closest object in the room bigger (figure 10c) or on a different baseline at the bottom of the page (figures 10f and 11a).

Figures 11a, b and c: Drawings by first grade pupils. Theme: my house

Figures 12a and b: Drawings of mazes by first grade pupils

Figures 12c and 13d: Drawings of maps by first grade pupils

The only difference of the drawings made by the second grade pupils that have been collected so far is that there is less color and that the vast majority of the mazes are drawn as strictly geometrical spaces.

The pupils of the third grade were the first to produce geographical maps. It is easy to understand the reason why such maps are firstly drawn at this age, as in the Greek educational system the subject of Geography is introduced in the third grade. Other than that, it is interesting to point out that the majority of the images are drawn using only pencil, while almost all of them use the bird-eye view combined with the centering technique. Geometrical shapes are widely used.

Figure 13: A drawing of a bedroom, using geometrical shapes, by a girl in the third grade

From the fourth grade onwards the concept of space seems to be successfully perceived in the children’s minds and implied in their drawings. Spatial depth (the notions of far and close) is visible. It would be interesting to find out whether children are taught of the concept of space or how to represent it in drawing in the school curriculum, but this is a theme for another research.

A very clear difference between the drawings made by the children living in the village and the children living in the big city was found to be the speed of spatial development. The easy explanation for that seems to be the different, wider curriculum offered to the children in the schools of the big cities. The enlarged zone program of the city schools offers to the children subjects like music, foreign language, PC learning, theatrical education and art. These classes, including the art class are required classes for all the children of the school. In the schools of the village Koufalia, on the other hand, the typical school program, which do not offer the above mentioned classes, but is strictly oriented around the study of the semiotic systems of language and mathematics—does not encourage artistic ability. When asked their opinion about drawing, children living in the village said that ―drawing is for small children‖—easy to understand why, as they stopped engaging with it as soon as they entered the first grade. On the contrary, children living in the big cities see drawing as a serious system of knowledge, like linguistics and mathematics. Consequently, the children living in the village were weak in capacity to draw space. Their lack of skill did not, however, mean lack of knowledge on the subject.

6.  Discussion

In all the drawings that I have gathered so far, the perception and representation of space is firstly experiential. The description of space is inseparable from the child’s experience in it. The drawings themed “my room” and “my house” describe the experience of the child’s usage of specific objects; the narrative is first and foremost experiential. Of course, these drawings are descriptive right from the outset. In the drawings of maps and mazes, however, the majority of children seem detached from personal experience.

All the children have already done big deductions: they have decided on scales, proportions and sizes, what to put into the frame of the picture and what to leave unseen, they have represented the three-dimensional world into the two dimensions on a piece of paper. Between the ages of 4 and 7, most children do not understand the concepts of a map or a maze and try to conceive them using mediated images, seen in television, school books or elsewhere.

For Piaget (1956) drawing is the tool to represent spatial conception. It was the practice of drawing that geometry was founded on, as Piaget quotes his teacher and philosopher of mathematics Léon Brunschvicg. Through their drawings during the years, children continuously revisit and reconstruct previous topological knowledge stemmed from cognition. Both artists and scientists start from experience and sensory perception, but after long periods of research, they arrive at aesthetic perception—that means deduction, abstraction, removal from experience, from the personal, the particular, the one and moving on to the universal, the collective, the unarguable truth. At some time, children are able to conceptualize metric relations, like direction (see the drawings of mazes) or distance. Wittmann writes that “according to Piaget, children have always had an implicit knowledge of what modern mathematicians could not conceptualize before the introduction of topology around 1900”.

Between the ages 7-12, children are able to apply projective geometry, symmetry (correspondence in size) and generally a clear and successful illusion of space in their drawings. If we follow the history of art, we will see that the techniques used to create space were invented in the fifteenth century, after the Renaissance period. During the research, I was surprised to find out some similarities of the stages of children drawing with the pre-history of human image-making. It is as if the child follows the same historical route in a few years. According to Marcussen (2008), almost all primitive art and, historically, all pre-Greek art basically have the metric structure at which the child invariably arrives.

Many well-known modern artists highly valued the simplicity of forms, the abstract thinking and free spatial orientation in children drawing. In 1912 Kandinsky wrote that the child has the natural ability to absorb the thing as such (Fineberg 1997:46). Kandinsky, as well as artists like Klee and Feininger were collectors of children’s drawings and they even used some of them as resources for their work. Kandinsky sought in children’s art to reveal an abstract, universal language.

If we examine children’s drawings with a Vygotskian lens like Brooks (2009) does, we will find that drawing can function as a mediator between a child’s spontaneous and scientific concept. For Vygotsky (1987) spontaneous concepts derive from direct encounter with things, experience, memory and increasing generalizations. See, for instance, through figures 1-4, how Christos started with drawing his family’s car and continued experimenting until he formed a satisfying for him sign for cars and then for racing cars. This procedure involved high mental functions, as Christos had to find the structural elements of cars, he had to make reductions, abstractions from the thing known through experience (the family’s car) and then make groupings and generalizations in order to imply through one drawing the notion of the whole kind (cars and specifically racing cars). His drawings imprinted his thinking route. Through his continuous drawings, he experimented on the notion of cars, from the specific to the general, and learned more things about the essence of cars. His newly acquired knowledge was communicated, again through his drawings to other people (his family, his classmates).

The same experience-memory-thinking-abstraction-grouping-generalization scheme is visible in the drawings of the children of the sample studied, as they grow from 4 to 12 years old. At young ages, they defined their room with its more functional element—a bed, for sleeping, or a desk, for studying. They went on to include in the drawing many personal things, what stemmed from their experience with the room—they placed the objects in their surroundings and examined relations, distances, orientation of objects as such and in relation with each other. This examination became crucial for their understanding of space and spatial representation. The older children removed the objects from the burden of personal associations (figure 15). This study from the thing as such to the thing as related to its surroundings and other things and then to the generalized thing requires high mental functions and is the exact procedure scientists and artists follow, as shown in previous research (Papadaki 2013).

Scientific concepts, on the other hand, as Vygotsky (1987) puts it, need the following of the opposite path: the drawing subject does not now go from the thing to the concept, but rather from the concept to the thing. In order to test this route, we asked children to draw maps and mazes, which, obviously, they had no experience with. Younger children were much influenced by mediated experience and tried to bring the concept closer to known things, e.g. maps of treasures, as seen in toys, books or television, maps as directions to go to specific places, as seen in children’s party invitations. The first drawings of mazes were exact copies of images seen somewhere, even if they were not exactly mazes—far from it. The last drawing attempts, however, as shown in figure 16, included a big percentage of architectural mazes and many geometrical influences. Going from the concept to the thing is a much more difficult task. For children living in big cities that have the chance to follow art classes in school, this process takes place faster and easier. It was puzzling to see perfect architectural mazes and geometrical shapes made from students of the second grade and third grade. These students were not yet or were hardly introduced to Geometry.

All children should be able to acquire competence in such a valuable tool for their cognitive development. As a meta-cognitive, deliberate, meaning-making activity, a perfect semiotic system that could be the base of a kandiskian universal language, children drawing involves high mental functions, entails discovering the essence of things and concepts and uncovers, in the same way as art and science do, important information about the world. Children’s drawing communicate the visible, but unseen.

References

ALLAND, A. (1983). Playing with form: children draw in six cultures, New York: Columbia University Press

ARNHEIM, R. (1954). Art and visual perception: a psychology of the creative eye.California: University of California Press

ARNHEIM, R. (1969). Visual Thinking. California: University of California Press

BROOKS, M. (2009). “What Vygotsky can tell us about young children drawing”. International Art in Early Childhood Research Journal. Vol 1. No 1. 

BRUNER, J. S. (1966). On cognitive growth, I and II. In J. S. Bruner, R. R. Oliver, P. M. Greenfield, eta/. (Eds.), Studies in cognitive growth ( pp. 1-67). New York, London, Sidney: John Wiley & Sons.

FINEBERG, J. (1997). The Innocent eye. Princeton University Press.

GOLOMB, C. (1992). The child’s creation of a pictorial world. California: University of California Press

GOODNOW, J. (1977). Children Drawing. Harvard University Press

HALL, E. (2009). Mixed messages: The role and value of drawing in early education.

International Journal of Early Years Education, 17(3), 179–190.

HARALAMBIDOU, P. (1998). ―The Fall, sketchbook‖, in Critical Architecture, ed. Rendell, J., Hill, J., Fraser, M., Dorrian, M., Oxon: Routledge

KELLOGG, R. (1970). Analyzing children’s art. Mayfield Pub Co

KRAMPEN, M. (1991). Children’s Coding: Iconic Coding of the Environment. New York: Springer Science and Business Media

KRESS, G. (2003). Literacy in the New Media Age. London: Routledge

LARKIN, J. H., & Simon, H. A. (1987). Why a diagram is (sometimes) worth ten thousand words. Cognitive Science, 11,65e99.

LEOPOLD, C. and Leutner, D. (2012). Science text comprehension: Drawing, main idea selection, and summarizing as learning strategies, Learning and Instruction, 22, 16-26 Luquet, G. H. (1927). Le dessin enfantin. Paris: Alcan.

MARCUSSEN, L. (2008). The architecture of space-The space of architecture. The Danish Architectural Press

MATTHEWS, J. (1984). Children drawing: Are young children really scribbling? Early Child Development and Care, 18 (1-2), 1-39

PAPADAKI, E. (forthcoming 2015) ―The semiotics of children drawings: A comparative study of art, science and children drawing‖. ebook Changing worlds & Signs of thetimes: Selected writings from the 10th International Conference on Semiotics, October 2013, Volos, Greece

PEIRCE, C. S. (1965-1966). Collected papers of Charles Sanders Peirce (Vol. I-IV). Charles Hartshorne, Paul Weiss, and Arthur W. Burks (Eds.). Cambridge: Harvard University Press.

PIAGET, J., & Inhelder, B. (1956). The Child's Conception of Space. New York: The HumanitiesPress Inc

SEBEOK, T. A. (1977). Ecumenicalism in semiotics. InT. A. Sebeok (Ed.), A peifusion of signs (pp. 180-206). Bloomington and London: Indiana University Press.

SPANKIE, R. (2009). Interior architecture: Drawing out the interior. Laussanne: AVA Publishing

VAN LEEUWEN, T. (1998). ―It Was Just Like Magic: A Multimodal Analysis of Children’s Writing‖, Linguistics and Education 10(3): 273–305.

VYGOTSKY, L. (1987). The collected works of L.S. Vygotsky. New York, NY: Plenum Press.

WITTMANN, B. ―Jean Piaget and the Child’s Spontaneous Geometry‖ in Meaningful Scribbles: An Epistemic History of Children’s Drawings, available http://www.mpiwg-berlin.mpg.de/en/news/features/feature11 (last visit 20/12/2014)